(a) 2x 3y = 12(i) and x y = 1(ii) (ii)×3 ==> 3x 3y = 3(iii) Now we can eliminate y by adding (i) & (iii) (i) (iii) ==> 5x = 15 so x=3Algebra Solve by Addition/Elimination xy=9 , 5x2y=32 x y = −9 x y = 9 , 5x − 2y = 32 5 x 2 y = 32 Multiply each equation by the value that makes the coefficients of y y opposite (2)⋅(x y) = (2)(−9) ( 2) ⋅ ( x y) = ( 2) ( 9) 5x−2y = 32 5 x 2 y = 32 SimplifyClick here👆to get an answer to your question ️ Solve the equation by substitution method 2x 3y = 9 , 3x 4y = 5

Solve By The Elimination Meth See How To Solve It At Qanda
3/x-1/y=-9 2/x 3/y=5 by elimination method
3/x-1/y=-9 2/x 3/y=5 by elimination method- y = 2 x = 1 We can solve this one of two ways;Share It On Facebook Twitter Email 1 Answer 1 vote answered by Tahseen Ahmad (301k points) selected by ShasiRaj Best answer Let 1/x = u and 1/y = v So, the given equations becomes




Solve 3 X Y 2 X Y 2 And 9 X Y 1 Brainly In
x y = 4, i 2x 3y = 9, ii from eq i, y = 4 x put the value of y in eq ii 2x 3( 4 x) = 9 2x 12 3x = 9 5x = 21 x = 21/5 put x =21/5 in y7 2x Y=4 x 4y=5 10 3x 5y6 12 3x 2y19 3x8y = 3x Y22 14 x 2y=10 15 15x3y 8 21x 3y10 X3y =10 Bjective 2 Multiply When Using Elimination Method Solve Each System By The Elimination Method Check Your Answers 17, 6x 7s=10 8xShare It On Facebook Twitter Email 1 Answer 0 votes answered by AmirMustafa (600k points) selected by Vikash Kumar Best answer The given equations are
x = 1 y = 2 Ok So prefer the elimination method, but you can do this with substitution as well First put the equations on top of each other x 2y= 5 2x3y=4 Then find the variable that would be easiest to cancel out I think it's x because you only have to modify one of the equations Let's multiply the first equation by 2 This will allow us to cancel out the two x's in theAnswer to Solve 2 x 3 y = 14 and 3 x 4 y = 4 by the method of elimination By signing up, you'll get thousands of stepbystep solutions toGet stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!
Steps for Solving Linear Equation 2x3y = 5 2 x 3 y = 5 Subtract 3y from both sides Subtract 3 y from both sides 2x=53y 2 x = 5 − 3 y Divide both sides by 2 Divide both sides by 29x – 2(9) = 108 x = 14 Answer x = 14 and y = 9 ← Prev Question Next Question → Solve for x and y 3/x 1/y 9 = 0 , 2/x 3/y = 5 Use the Substitution method to solve the system of equations y 2x = 5 3y x = 5 Solve one of the equations for x or y Let's solve the first one for y y 2x = 5 y = 2x 5 Now let's substitute 2x 5 for y in the second



1




Solve By Elimination Method 1 X 3y 7 And X Y 5 2 8x 5y 9 And 3x 2y 4 3 3x Y 3 And 4x Y 8 Brainly In
First, you have to equate the coefficients of the y so equation 1 will be doubled 4x 2y = 0 x 2y15/x y 5/x y = 2 Join / Login mathsAdvertisement Remove all ads
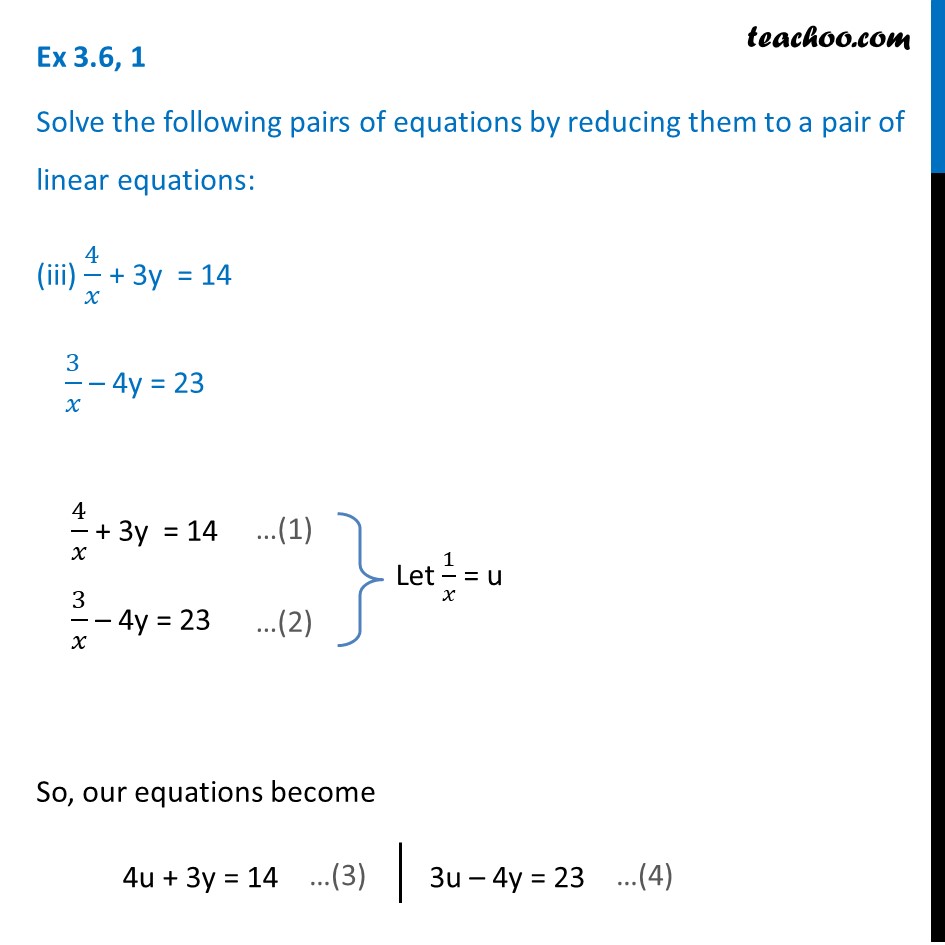



Ex 3 6 1 Iii Iv 4 X 3y 14 3 X 4y 23 Ex 3 6



Solve For X And Y X 2 Y 9 6 X 7 Y 3 5 Sarthaks Econnect Largest Online Education Community
Solve the Following Systems of Equations `3/X 1/Y = 9` `2/X 3/Y = 5` CBSE CBSE (English Medium) Class 10 Question Papers 6 Textbook Solutions Important Solutions 3111 Algebraic Methods of Solving a Pair of Linear Equations Substitution Method video tutorial ; 2/x 3/y = 5 pair of linear equations in two variables;In order to find the answer you need to solve this as a system of equations 2x y = 1 x 2y = 5 Solve for x in the second equation x = 5–2y Now substitute for x in the first equation 2(5–2y) y = 1 10–4y y = 1 10–3y = 1 Subtract 10 from bo




Ex 3 3 1 Solve By Substitution Method I X Y 14 Ex 3 3




Gauss Elimination
An example of a system of two linear equations is shown below We use a brace to show the two equations are grouped together to form a system of equations {2x y = 7 x − 2y = 6 A linear equation in two variables, such as 2x y = 7, has an infinite number of solutions Its graph isSolve by Addition/Elimination x2y=3 2x3y=9 x − 2y = 3 x 2 y = 3 2x − 3y = 9 2 x 3 y = 9 Multiply each equation by the value that makes the coefficients of x x opposite (−2)⋅ (x−2y) = (−2)(3) ( 2) ⋅ ( x 2 y) = ( 2) ( 3) 2x−3y = 9 2 x 3 y = 9 Simplify Tap for more steps Simplify ( − 2) ⋅ ( x − 2 y 3/x 1/y 9 = 0, 2/x 3/y = 5 linear equations in two variables;




Ncert Solutions For Class 10 Maths Chapter 3 Exercise 3 4 Pair Of Linear Equations In Two Variables




Nms 2nd Lesson Ii
Solve by Addition/Elimination xy=3 , xy=7 x y = 3 x y = 3 , x y = 7 x − y = 7 Multiply each equation by the value that makes the coefficients of x x opposite x y = 3Elimination method x2y=2x5, xy=3 \square!Solve the Following Pair of Linear (Simultaneous ) Equation Using Method of Elimination by Substitution 2( X 3 ) 3( Y 5 ) = 0 5( X 1 ) 4( Y 4 ) = 0




Solve Each Of The Following System Of Equations By Elimination Method I X Y 8 2x 3y 1 Ii Maths Linear Equations In Two Variables Meritnation Com




Solve X Y 7 And 3x 2y 11



0 件のコメント:
コメントを投稿